Various dissections of polyominoes and polygons
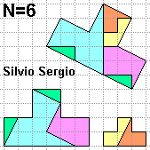
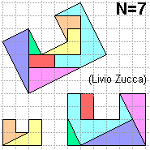
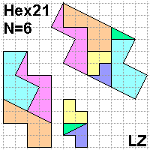
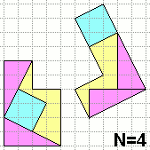
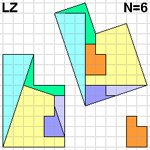
Starting from december 2009, Livio Zucca has proposed and discussed the following problem with the friends of the newsgroup it.hobby.enigmi: given a polyform or a polygon of side 1 and its double of side 2, dissect them in pieces that can be rearranged to form the corresponding figure of side sqrt(5) (since 1+4=5).
Livio has also considered the problem of dissecting a pentomino and rearranging the pieces to form a tetromino or the domino.
Clearly these problems are always solvable, but we are interested in finding the minimal number of pieces. You can see the results obtained until now by clicking on the images below.
 |
 |
 |
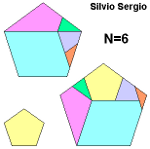 |
| 1,2,3,4-ominoes | pentominoes | hexominoes | polygons |
 |
 |
||
| pento-tetro | pento-domino | 1+3=√10 |

