A cube, which has the edge equal to square root of 10, has an area of:

SQR(10) * SQR(10) * 6 = 60

The twelve pentominoes also. Now we try a solution at least. And this is a solution:

You could check the surface in the following picture. The pentominoes has been inclined of arctan(1/ 3) and refolded on the edges. I don't know how many solutions have the problem. If anyone knows it or knows how to calculate it, please send me an e-mail.

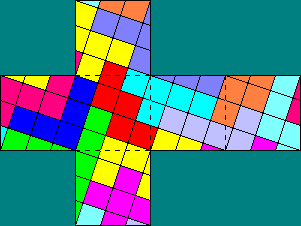
I greatly enjoy seeing the pentominoes cover a closed surface! Imagine, now, to blow up the cube like a balloon, a sphere. This also will be covered with pentominoes. But we must tame the definition, not 'Squares', but 'Quadrangular Segments of Spherical Bowl'. (like a field on the Earth). Personally I'll paint a soccer ball like the picture below.

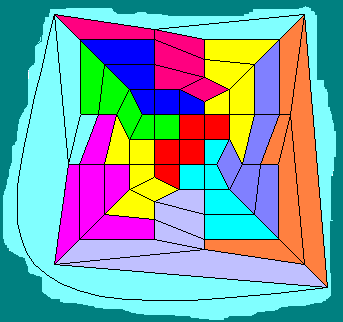
The next picture tells the same things, but it's a grapho. It symbolizes the edges of a polyhedron with 60 quadrangular faces. This polyhedron has 62 vertices, 54 with 4 edges and 8 with 3 edges. The edges are obviously 120. The 60th quadrangle has the same perimeter of the grapho and it's closed to infinity. You could recognize the deformed twelve pentominoes in the picture.

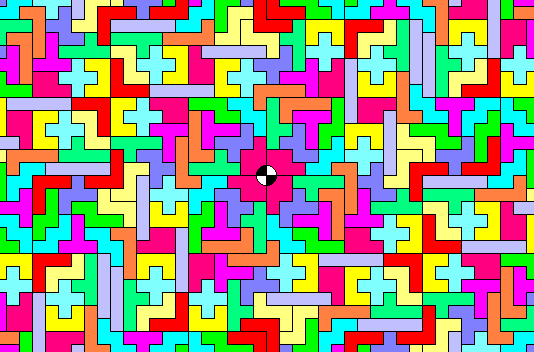
With regard to infinity, I ask: could the twelve pentominoes tile the plane, without constitute rectangles or separation straight lines? The answer is yes. And each pentomino has his symmetric.