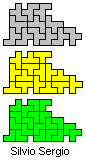
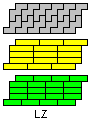
Pento-Tetro-Trominoes
Livio Zucca

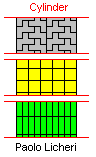
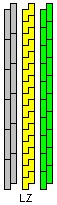
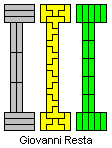
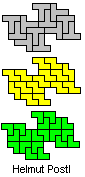
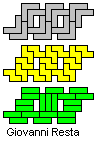
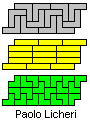
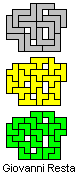
We are searching for the 120 shapes that can be covered by a pentomino or a tetromino or a tromino. We'll give precedence to the solutions on the finite plane with the smallest surface. If there aren't solutions on the plane, we'll accept solutions on cylindrical surface or on Moebius strip. Solutions on torus are not interesting because each pentomino, tetromino and tromino covers a torus. If you have better solutions, please send me HERE with gif, jpg, bmp, FidoCad or ascii-art format.
| F5I4I3 | F5L4I3 | F5N4I3 | F5Q4I3 | F5T4I3 |
 |
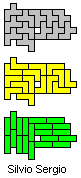 |
 |
 |
 |
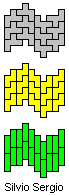
| F5I4L3 | F5L4L3 | F5N4L3 | F5Q4L3 | F5T4L3 |
 |
 |
 |
 |
 |
| I5I4I3 | I5L4I3 | I5N4I3 | I5Q4I3 | I5T4I3 |
 |
 |
 |
 |
 |
| I5I4L3 | I5L4L3 | I5N4L3 | I5Q4L3 | I5T4L3 |
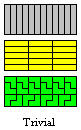 |
 |
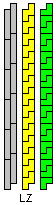 |
 |
 |
| L5I4I3 | L5L4I3 | L5N4I3 | L5Q4I3 | L5T4I3 |
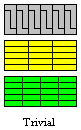 |
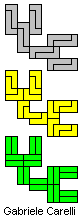 |
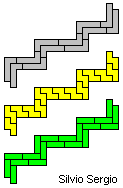 |
 |
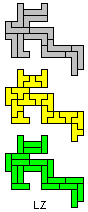 |
| L5I4L3 | L5L4L3 | L5N4L3 | L5Q4L3 | L5T4L3 |
 |
 |
 |
 |
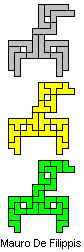 |
| P5I4I3 | P5L4I3 | P5N4I3 | P5Q4I3 | P5T4I3 |
 |
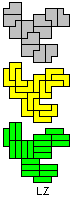 |
 |
 |
 |
| P5I4L3 | P5L4L3 | P5N4L3 | P5Q4L3 | P5T4L3 |
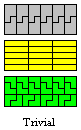 |
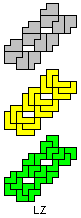 |
 |
 |
 |
| N5I4I3 | N5L4I3 | N5N4I3 | N5Q4I3 | N5T4I3 |
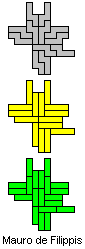 |
 |
 |
 |
 |
| N5I4L3 | N5L4L3 | N5N4L3 | N5Q4L3 | N5T4L3 |
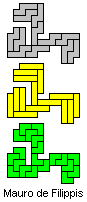 |
 |
 |
 |
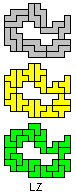 |
| T5I4I3 | T5L4I3 | T5N4I3 | T5Q4I3 | T5T4I3 |
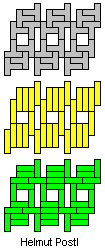 |
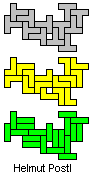 |
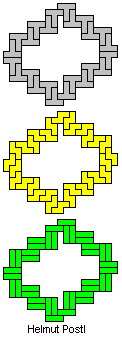 |
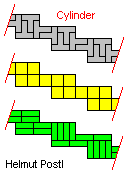 |
 |
| T5I4L3 | T5L4L3 | T5N4L3 | T5Q4L3 | T5T4L3 |
 |
 |
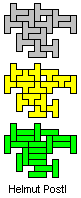
 |
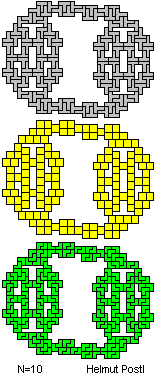 |
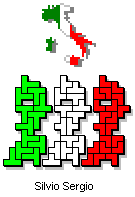 |
| U5I4I3 | U5L4I3 | U5N4I3 | U5Q4I3 | U5T4I3 |
 |
 |
 |
 |
 |
| U5I4L3 | U5L4L3 | U5N4L3 | U5Q4L3 | U5T4L3 |
 |
 |
 |
 |
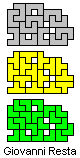 |
| V5I4I3 | V5L4I3 | V5N4I3 | V5Q4I3 | V5T4I3 |
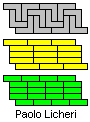 |
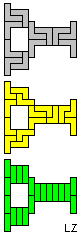 |
 |
 |
 |
| V5I4L3 | V5L4L3 | V5N4L3 | V5Q4L3 | V5T4L3 |
 |
 |
 |
 |
 |
| W5I4I3 | W5L4I3 | W5N4I3 | W5Q4I3 | W5T4I3 |
 |
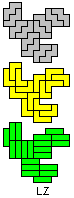 |
 |
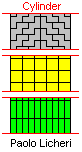 |
 |
| W5I4L3 | W5L4L3 | W5N4L3 | W5Q4L3 | W5T4L3 |
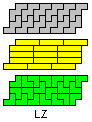 |
 |
 |
 |
 |
| X5I4I3 | X5L4I3 | X5N4I3 | X5Q4I3 | X5T4I3 |
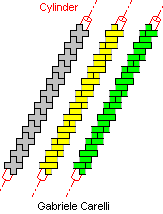 |
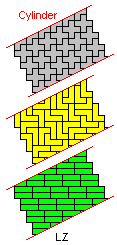 |
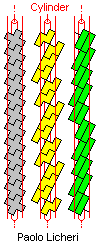 |
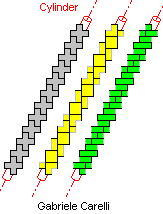 |
 |
| X5I4L3 | X5L4L3 | X5N4L3 | X5Q4L3 | X5T4L3 |
 |
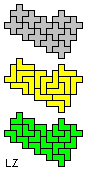 |
 |
 |
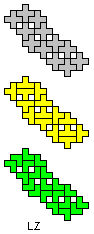 |
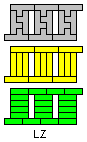
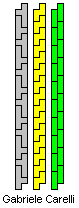
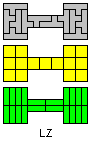
| Y5I4I3 | Y5L4I3 | Y5N4I3 | Y5Q4I3 | Y5T4I3 |
 |
 |
 |
 |
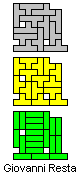 |
| Y5I4L3 | Y5L4L3 | Y5N4L3 | Y5Q4L3 | Y5T4L3 |
 |
 |
 |
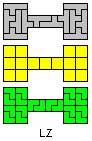 |
 |
| Z5I4I3 | Z5L4I3 | Z5N4I3 | Z5Q4I3 | Z5T4I3 |
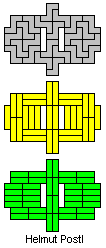 |
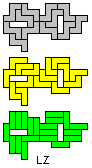 |
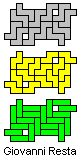 |
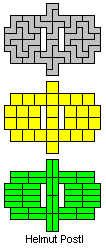 |
 |
| Z5I4L3 | Z5L4L3 | Z5N4L3 | Z5Q4L3 | Z5T4L3 |
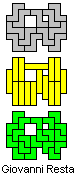 |
 |
 |
 |
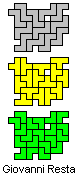 |

See also:
Pento-tro-dominoes
Tetrominoes Challenge
LINK:
Visit the wonderful site of Jorge Luis Mireles
_________________
It isn't trivial!
First edition: Dic 7th, 2003 - Last revision: Dic 29th, 2003




 Index
Index




